An interdisciplinary conference (pure and applied maths, physics) at CIRM 23 - 27 May 2022
Link: https://conferences.cirm-math.fr/2225.html
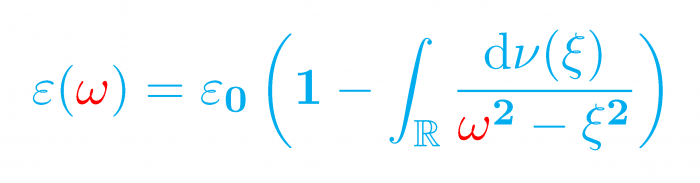
Description
Herglotz-Nevanlinna functions are analytic functions with a nonnegative imaginary part in a given tubular domain (such as the upper-half plane of the complex plane). Discovered in the 1920s, these functions have a long history in analysis (spectral theory, moment problem...) and appear more recently as a key tool in applied sciences to study the dispersion in electromagnetic systems such as metamaterials, in the analysis of effective tensors of composite materials and in the study of the Dirichlet-to-Neumann (DtN) map in inverse problems. Indeed, the mathematical properties of Herglotz-Nevanlinna functions (integral representations, sum rules, continued fraction expansions...) are extremely useful to derive fundamental limits and quantitative bounds on a physical property with respect to the frequency (for dispersive systems) or with the respect to the geometry (for composite materials). Knowing the sharpness of these bounds in a class of materials generates a lot of applications in electromagnetism and composites design. Finally, a recent breakthrough appears in inverse problems and imaging in composite media where a deep connection was made between the DtN map and these functions. However, nowadays, most of the topical issues necessitate a deep understanding of Herglotz-Nevanlinna’s functions depending not only of one variable but also of several variables (as for composites composed of at least three phases or spatially dispersive media whose permittivity depends both on the wave number and the frequency). Another challenge which appears to be crucial for some applications (e.g. the DtN map in composite media) is to deal with Herglotz-Nevanlinna operator valued functions. Thus, the aim of this conference is to gather several communities: pure mathematicians, applied mathematicians, physicists and engineers around Herglotz-Nevanlinna functions and their connections with physics and engineering. Its main focus is communicating the needs of people on the applied side and the tools of those on the more theoretical side and thus laying the foundations for future interactions.
Contact: If you are interested to attend the conference, please contact Maxence Cassier (Institut Fresnel, cassier@fresnel.fr) or Boris Gralak (Institut Fresnel, gralak@fresnel.fr).